Sparked by a conversation this past weekend about the usefulness of the half-angle identities, I constructed geometric proofs for and
. Since I’ve never seen these anywhere before, I thought I’d share.
And while I was at it, I thought I’d share all my other geometric proofs, so here they are, posted mostly without comment.
Some of these are so well-known as to be not worth mentioning. Many of them have been stolen from Proofs Without Words I or Proofs Without Words II. I came up with a few of them myself. Frustratingly, almost none of them are to be found in Precalculus textbooks, where they might be learned and appreciated.

________________________________________________________

__________________________________________________________

_____________________________________________________________

________________________________________________________________

_______________________________________________________________
______________________________________________________________
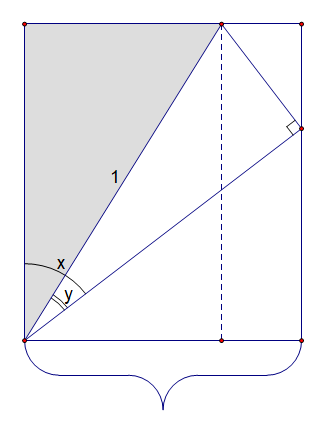
_________________________________________________________________

______________________________________________________________
Though this one is my favorite:

____________________________________________________________________________

_______________________________________________________________________________________
Partially because of the way it naturally generalizes into the proof of the derivative of sine. If you just let approach 0,
approaches 1 and that point in the interior of the circle ends up on the circle, where
merges with
itself.

_____________________________________________________________________________________

______________________________________________________________________________________

_______________________________________________________________________________________

___________________________________________________________________________________

__________________________________________________________________________________

___________________________________________________________________________________________

____________________________________________________________________________________
 ____________________________________________________________________________________
____________________________________________________________________________________
And finally, one that shows that the sum of a sine and cosine function of the same argument is also a sinusoid. Since I lost the original picture and don’t feel like remaking it, you’ll have to complete the proof on your own!

____________________________________________________________________________________
Update: After some feedback on twitter, I’ve decided to add a few more diagrams. Tim Brzezinski sent me a link to his website of geometric proofs of trig identities and he had some that I’ve never seen before.
Check it out!
https://www.geogebra.org/m/DxAcj8E2#material/QedMT7Pw
I’ve taken two of his diagrams and added them below.

_________________________________________________________
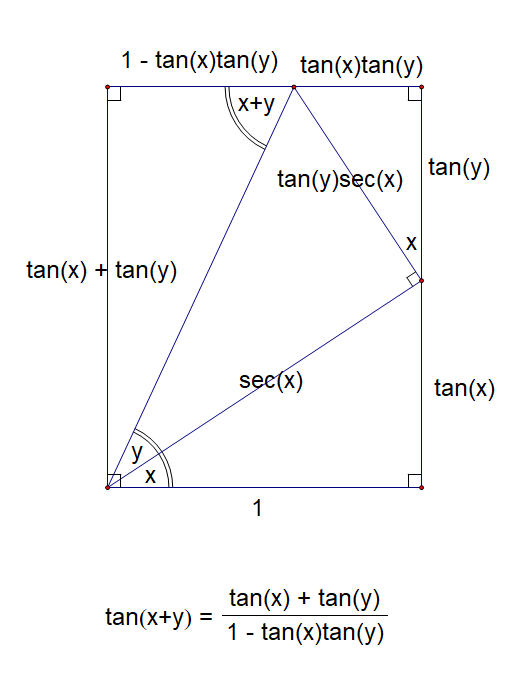
____________________________________________________________________________________

_________________________________________________________________________________________


These are beautiful, Will Rose!! 😀
I have some proofs, are they known?
Link: https://geometriadominicana.blogspot.com/2020/06/geometric-proof-of-sum-angle-formula.html
Geometrifying Trigonometry is a formal language structure which generates these picture proofs and also conjectures from these automatically from given single identity. Geometrifying Trigonometry generates constructions protocols and also says how many line segments overlap and which points are common to which lines
Pingback: 三角恆等式的幾何證明 – 嘎嘎酷